

In this case, we can use areas to explain how to complete the square. Where both \(a\) and \(b\) are positive numbers. Let's say we want to solve an equation of the form When completing the square, you rewrite a quadratic equation to the form \((ax+b)^2=c\). You can also easily solve equations of the form \((ax+b)^2=c\), first take the square root of both sides, then solve for \(x\).

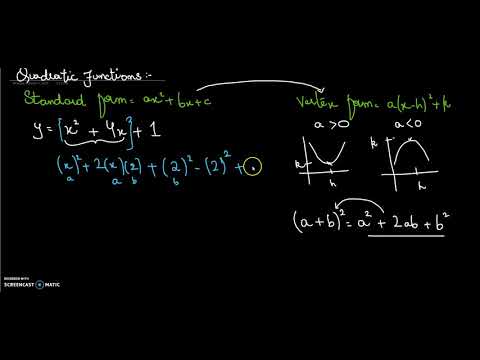
It is easy to solve a quadratic equation of the form \(x^2=c\), you just take the square root of both sides. Completing the squareĬompleting the square is a method used for solving quadratic equations and for rewriting quadratic functions to vertex form. Given that this passes through the point (1,2), we must have: 2 a(1 3)2 +4 4a +4. In our example the vertex (h,k) is (3,4), so we can write: f (x) a(x 3)2 +4. where (h,k) is the vertex and a is a constant multiplier. In order to rewrite a quadratic function from general form to vertex form, you must know how to complete the square. A quadratic function can be written in vertex form as: f (x) a(x h)2 +k. The graph of a quadratic function, or the correspondence between the graph and solutions to quadraticĮquations, two other forms are more suitable: vertex form Try out the practice question to test your understanding.The most common way to write a quadratic function is to use general form: Hence, the coordinates of the vertex of this quadratic equation is (1, 1). Hence, the y-coordinate of the vertex is 1. To graph a parabola using vertex form, we first graph the vertex point. See the image above for a parabola graphed with the vertex labeled. In other words, for the vertex, (x, y) (h, k). Now, we can use this x-coordinate to find the y-coordinate of the vertex. When graphing a quadratic function with vertex form, the vertex’s x and y values are h and k respectively. Let's change back to the original equation. With this, the vertex has the x-coordinate of 1. Knowing this, we can substitute 'b' with 2 and substitute 'a' with -1. Hence, we can see that, 'a' is equals to -1, ‘b’ is equals to 2, and 'c' is equals to 0. To find the values of 'a' and 'b', we can compare this equation with the general equation, y = ax^2 + bx +c.įor easier comparison, we can rewrite this quadratic equation as, y = -1x^2 +4x + 0. Let's find the coordinates of this vertex.Īgain, we start with the formula for the x-coordinate of the vertex of a quadratic equation, x = -b/2a. Now, the vertex is located at the highest point on the graph. The graph for this equation is shown here. Consider the quadratic equation, y = -x^2 +2x. To find the y-coordinate of the vertex, simply plug the value of -b / 2a into the equation for x and solve for y. Hence, the vertex of the equation has the coordinates of (2, -5). How do you find the vertex of the quadratic function We find the vertex of a quadratic equation with the following steps: Get the equation in the form y ax2 + bx + c. Hence, the vertex has y-coordinate of -5. Adding -16 with 3, gives -13.Īgain, we can substitute x with 2. Now, we can use the x–coordinate to find the y-coordinate of the vertex. With this, we know that the vertex of the quadratic equation has the x-coordinate of 2. 8 divide by 4, gives 2įinally, we have x equals to 2. Knowing this, we can substitute 'b', with -8, and substitute 'a', with 2. The vertex of a quadratic equation of parabola is nothing but the highest or the lowest point of the quadratic equation. To find out the values of 'a', 'b' and 'c', we can rewrite this equation as, y = 2x^2 + (-8)x +3.īy comparing this equation with the general equation, we can see that, 'a' is equals to 2, 'b' is equals to -8, and 'c' is equals to 3. Let's see an example on using this formula, by using this equation, y = 2x^2 -8x +3. Where, 'a', 'b' and 'c' are the coefficients for each term respectively. The general equation of a quadratic equation is given as, y = ax^2 +bx, +c. Now, what are 'a' and 'b'? Let's find out. Now, there is a formula to calculate the x-coordinate of the vertex of a quadratic equation.

Hence, this point is the vertex of a quadratic equation.Īlso, if you have a quadratic graph as shown here, the lowest point, is also the vertex of the quadratic equation, y = 2x^2 -8x +3. Notice that, this is the highest point on the graph.
Now, this is the graph of the quadratic equation, y= -x^2 +2x. In this lesson, we will learn about the vertex of a quadratic equation.


 0 kommentar(er)
0 kommentar(er)
